Mathematics
Intent
Why do we teach mathematics, and why is it so important? Because the habits of thinking mathematically are life-enriching. Because it is vital to be numerate to participate fully in society and democratic processes. Because it is essential to everyday life, critical to science, technology and engineering, and necessary for financial literacy and most forms of employment. Because our economy depends on a numerate workforce and specialists in maths and science related disciplines.
So, when we talk about the ‘intent’ of mathematics at Woodside Junior School, it is to ensure that every young person, regardless of background, has a rich and meaningful maths education that will provide them the foundation required for understanding the world and succeeding in secondary school and beyond.
At Woodside, we use the National Curriculum as our foundation and use White Rose Maths, supplemented by other curriculum resources, to deliver a carefully sequenced curriculum. Our aim is that all pupils:
- Become fluent in the fundamentals of mathematics, including through varied and frequent practice with increasingly complex problems over time, so that pupils develop conceptual understanding and the ability to recall and apply knowledge rapidly and accurately.
- Reason mathematically by following a line of enquiry, conjecturing relationships and generalisations, and developing an argument, justification or proof using mathematical language
- Can solve problems by applying their mathematics to a variety of routine and non-routine problems with increasing sophistication, including breaking down problems into a series of simpler steps and persevering in seeking solutions
We follow a mastery approach to mathematics but are aware that many different interpretations of ‘mastery maths’ exist. So what does mastery maths mean to us at Woodside?
- Curriculum coherence: The curriculum is designed to enable a coherent learning progression.
- It is the belief that with good teaching, appropriate resources, a carefully sequenced curriculum and ‘can do’ attitude, all children can achieve in and enjoy mathematics.
- Teaching is focused, rigorous and thorough, to ensure that learning is sufficiently embedded. Long term gaps in learning are prevented through speedy teacher intervention in lessons.
- More time is spent on teaching topics to allow for the development of depth and sufficient practice to embed learning.
- It is a teaching and learning approach that aims for pupils to develop deep understanding of maths rather than being able to memorise key procedures or resort to rote learning. Take finding a fraction of an amount as an example. In the past, teachers may have taught pupils to ‘divide by the denominator and multiply by the numerator.’ This required pupils to remember a procedure, rather than fully understanding the processes behind it. Instead, teachers carefully select representations of mathematics to help pupils to ‘see’ the maths. These representations become mental images that pupils can use to think about mathematics, supporting them to achieve deep understanding.

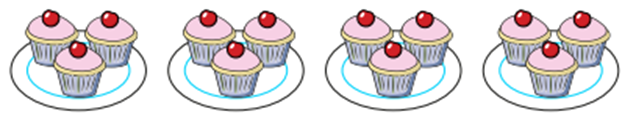
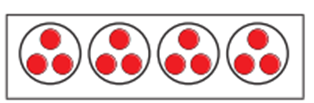
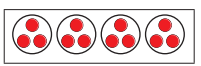 There are 4 plates.
There are 4 plates.
Each plate has 3 cakes.
There are 4 equal groups of 3.
4 x 3 = 12
This is the same as 3 + 3 + 3 + 3
- Fluency: Efficient, accurate recall of key number facts and procedures is essential for fluency, freeing pupils’ minds to think deeply about concepts and problems. Time is given throughout the curriculum for pupils to rehearse core facts, methods and strategies that can then be used to complete exercises and solve problems. We want our pupils to commit key facts to memory. Examples include rehearsing and practising number bonds, times tables, division and multiplying and dividing by 10, 100 and 1000.
- The end goal and expectation is for all pupils to have acquired the fundamental facts and concepts of maths for their year such that by the end of each year, they have achieved mastery in the maths they have been taught-so their learning is sustainable over time and can be built upon in subsequent years. At this point they are ready to move confidently on to their next stage of maths.
- Mastery of a mathematical concept means a child can use their knowledge of the concept to solve word problems and undertake complex reasoning using the appropriate mathematical vocabulary. Fluency frees up the working memory so that pupils are able to recognise relationships, make connections and choose appropriate methods and strategies to solve problems. Mastery means being able to use one’s knowledge appropriately, flexibly and creatively and to apply it in new and unfamiliar situations.
For example:
Year 3

Year 4
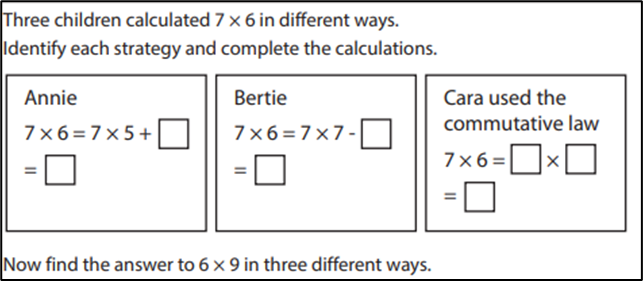
Year 5

Year 6
Last month Kira saved 3 5 of her £10 pocket money. She also saved 15% of her £20 birthday money. How much did she save altogether?
- Mathematical thinking is central to how pupils learn mathematics and includes looking for patterns and relationships, making connections, conjecturing, reasoning and generalising. All pupils are actively encouraged to engage in mathematical thinking in lessons, communicating their ideas and using precise mathematical language.
- Regular assessment to check for understanding and address any gaps or misconceptions.
- Pupils will have achieved mastery of the curriculum when they have sufficient depth of knowledge and understanding to reason and explain mathematical concepts and procedures and are able to use them to solve a variety of problems.
Our curriculum is taught in an order that helps pupils to build on their existing skills and knowledge. Our skills progression very clearly shows how skills and knowledge develop over time as they move through KS2. For example:
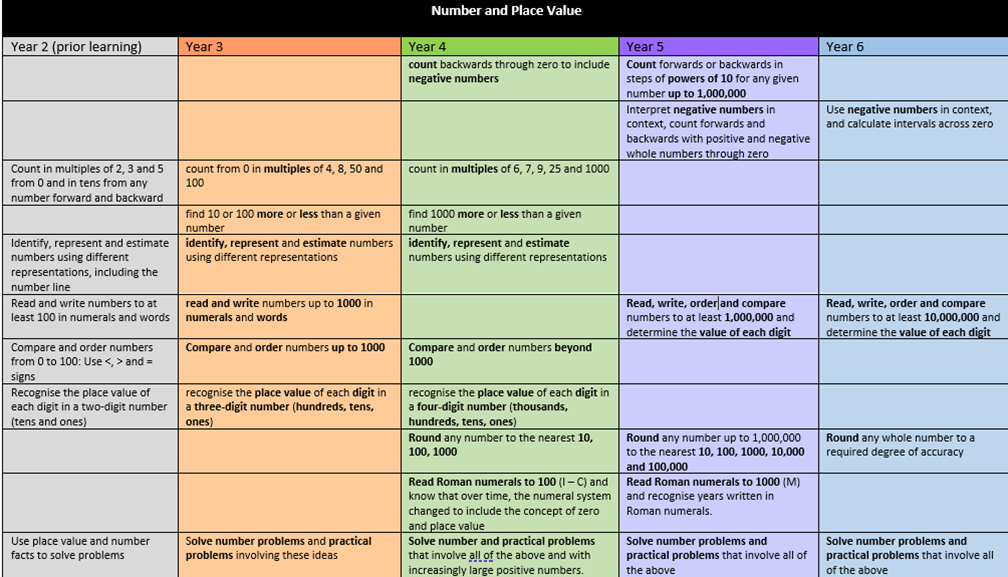
This is also the case within each year group. For example, in year 5 pupils build on their knowledge of multiplication and division from year 4; then use their knowledge to learn about fractions; then use this knowledge of fractions to learn about decimals and percentages.
Pupils with SEND access lessons alongside their peers. Teaching is adaptive and responsive to the needs of these pupils. LSAs are deployed effectively to work with different children and groups, including those with SEND. Pupils may be supported with concrete or pictorial representations for longer, although we encourage all pupils to not overly-rely on physical aids. As with all children in a class, the teacher will use ongoing assessment-including within lessons- to determine whether these pupils need additional explanation, pre-teaching, resources or time to complete tasks.
More able: We recognise that some pupils will grasp concepts more rapidly than others and need to be stimulated and challenged to ensure continued progression. These pupils benefit from more enrichment and deepening of content, rather than acceleration into new content. Acceleration is likely to promote superficial understanding, rather than the true depth and rigour of knowledge that is a foundation for higher mathematics. Our pupils, whilst being taught the same curriculum content and objectives, are given further opportunities to enrich their understanding. For example:
Year 3
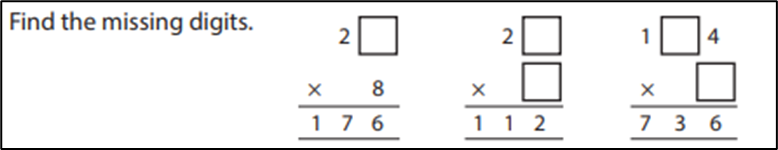
Year 4
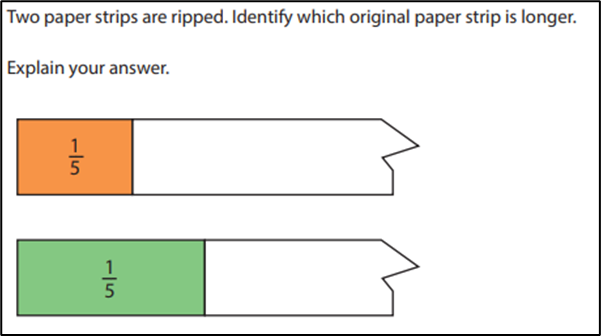
Year 5
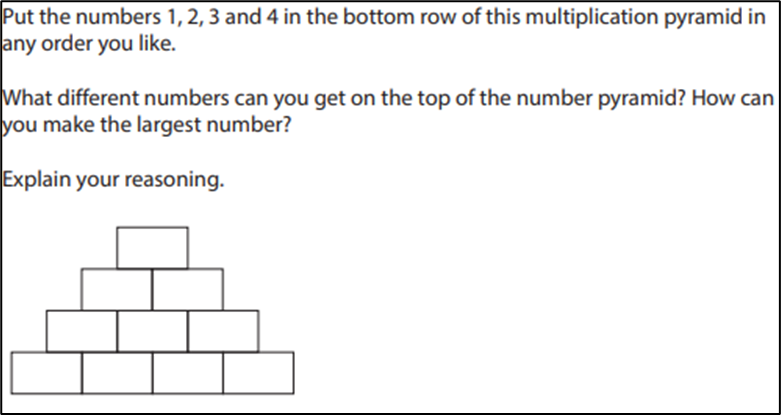
Year 6
Implementation
Our mathematics curriculum is taught by knowledgeable staff, and we dedicate one CPD session per half-term to developing teacher practice. The curriculum is taught in such a way that our pupils can build on their prior knowledge: From year to year, unit to unit and lesson to lesson. Individual lessons are planned to ensure that each segment builds upon the previous to advance more complex learning by the end of the lesson. All classes use working walls to display current learning and vocabulary. We follow the White Rose long-term overview and use their lessons and resources, although we supplement this with a number of additional resources to meet the needs of our pupils. We ensure that all pupils, regardless of ability, are exposed to fluency as well and reasoning and problem-solving.
Teachers check for understanding throughout the lesson using a range of techniques, for example, questioning, showing answers on mini whiteboards, over the shoulder marking, communication with the class LSA, mini quizzes and retrieval. This in-lesson monitoring is a key component of our mastery approach- to ensure that errors and misconceptions are picked up and dealt with swiftly so that all children master the lesson or group of lessons.
Staff at Woodside use a range of retrieval strategies in all curriculum subjects, and this is mirrored in maths. In all lessons, teachers find opportunities to elicit understanding of what has already been taught and use this information to provide feedback or adapt lessons where necessary. Retrieval is also a key component of our teaching and learning approach as we know that recall activities help the learning to ‘stick’ in their long-term memories.
Teachers also use pre-assessments at the beginning of each unit to check for understanding of what has been previously learnt. This enables teachers to check that the pupils have the prior knowledge needed to tackle the new learning. Teachers also use this as a starting point for new learning. Whilst all children then continue to learn the same content and objectives- as a part of our commitment to high aspirations for all-this provides teachers with the information they need to plan scaffolding or challenge where necessary.
We know how important it is for children to be given time to practice their efficient and accurate recall of key number facts. In the lower school, children are first taught about multiplication and division, what this ‘looks like’, how to work them out, and how they are related using concrete and pictorial representations. They are then given time to practice their times tables and related division facts so that by the end of year 4 they know these by heart and can answer with speed and accuracy. The children also use ‘TT Rockstars’ and are taught rolling numbers. Rolling numbers is a memorable and fun way to learn the times tables through ‘skip counting’ and allows children to be able to ‘work out’ their times tables by ‘counting on’.
The concrete - pictorial- abstract (CPA) approach to the teaching and learning of maths is a key component of our lessons. For example, children will first learn to add, subtract and exchange using dienes apparatus and place value counters before moving on to using pictorial representations. After this, they will then be able to solve calculations in the abstract. For example:

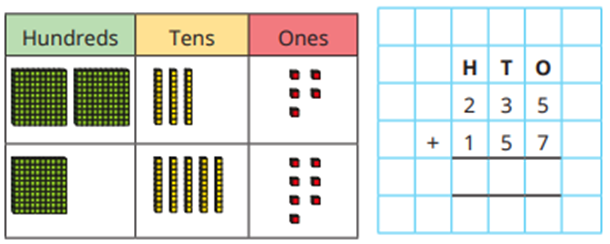
The concrete and pictorial stages are designed to help the children understand the abstract. They are shown side-by-side so that children make explicit links between the concrete-pictorial and abstract.
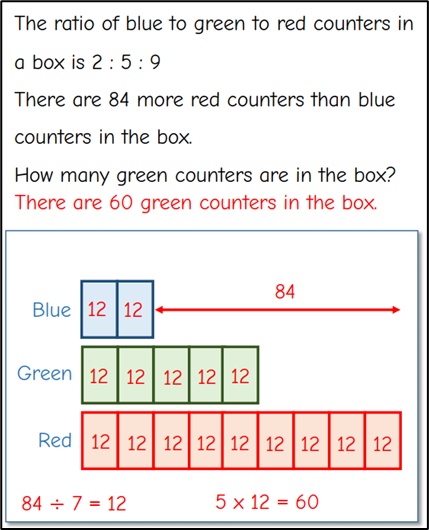
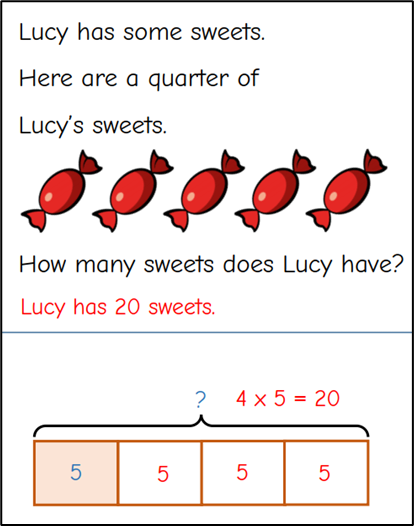
Similarly, our maths curriculum incorporates bar models. Bar models give a pictorial model of the underlying structure of a mathematical problem. There are many different types of bar model, and they can help illustrate basic concepts and solve basic problems. Bar models expose the mathematical concept, allowing children to more easily understand what calculation to perform.
Teachers give pupils opportunities to self- direct their learning in maths. For example, following the input, pupils may feel confident in their understanding of the key content and may choose to move to a deeper learning task. Teachers and LSAs work closely together and support groups of children in lessons to ensure that all children reach the desired outcomes. In lessons, children work as a whole class, in pairs, collaboratively and independently. At the end of each term (3 times per year) teachers use summative assessments to check that what has been taught has been learned and remembered. These take place two or three weeks before the end of term- allowing for teachers to go back and address any gaps in learning.
During lessons, pupils are expected to mark their own work after each section. They go back and correct errors but if they continue to find the questions difficult, will ask for help. This approach enables children to identify their own misconceptions and errors; ensures that pupils do not complete ‘whole chunks’ of learning incorrectly; enables misconceptions to be identified quickly; and gives pupils the opportunity to move onto deeper learning.
The subject lead visits lessons, delivers CPD and carries out book and planning scrutiny as well as pupil voice to ensure a continual development of the subject. World Maths Day is celebrated to hook children into maths in a fun and exciting way, with pupils given the opportunity to work collaboratively on stimulating investigations. Parent workshops are also planned for this year to further their knowledge of the curriculum and give them the tools needed to support learning at home.
Impact
Pupils at Woodside enjoy maths. They work with number confidently using a range of representations. They have accurate recall of number facts and secure knowledge of their times tables. The knowledge they gain enables them to say ‘I know that…’ as well as ‘I know how to…’ Pupils are able to solve problems, reason mathematically and use mathematical language to explain their thinking in a range of contexts. Pupils can apply their knowledge of maths across the curriculum (where appropriate) and attainment is consistently in the top 20% of schools nationally at the end of KS2. Fundamentally, our pupils leave here with the knowledge, skills and foundation they need to confidently move onto their next stage of learning at secondary school.

